

Much progress has been achieved by this approach, especially by Kronmüller et al. Micromagnetism tries to discuss the behavior of magnetic materials without detailed knowledge of the basic origin of magnetic ordering and anisotropy. In the case of RE–3 d (RE=rare-earth) intermetallics a phenomenological separation into the contributions of the RE and the 3 d sublattice is often meaningful. A simplified approach is the molecular field calculation. A rigorous calculation of the MCA from first principles is at present not possible. The lattice symmetry is therefore responsible for the MCA. Anisotropy, however, is the result of the interaction of the electron orbitals with the potentials of the lattice. The nature of this exchange is isotropic. e., the combination of the electrostatic coupling between electron orbitals and the Pauli exclusion principle. Long-range spin ordering over macroscopic distances is the result of exchange, i. Kirchmayr, in Encyclopedia of Materials: Science and Technology, 2001 4 Microscopic Origin of Magnetocrystalline Anisotropy
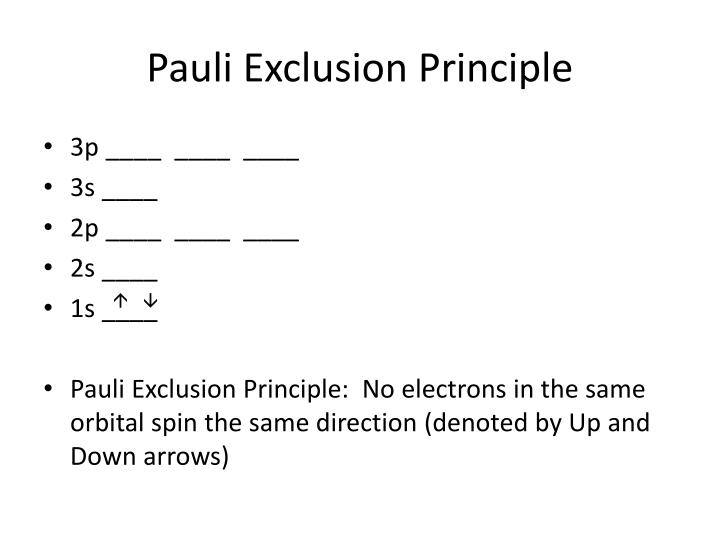
However, if the unpaired electrons are parallel to the same direction, the particles exhibit magnetic moment. If they exhibit random movement ( Figure 46(iii–v)), there will be no net magnetic moment. The overall magnetic activity depends upon the alignment of their unpaired electrons.
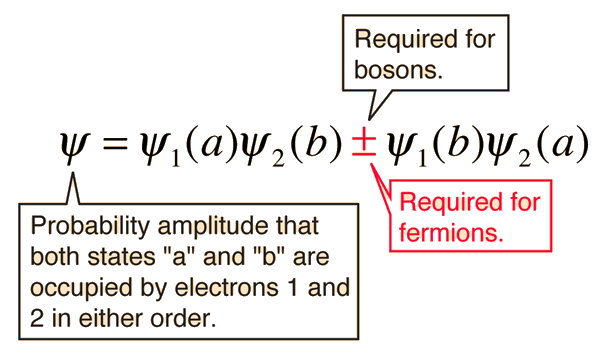
Atoms with unpaired electrons spinning in the same direction contain net magnetic moments and are weakly attracted to magnets. Although electron spin generates magnetic momentum, the opposite spins of the two electrons in the same orbital cancel out their magnetic momentum with no residual magnetic momentum. In certain cases, such as hydrogen atom or certain isotopes, nuclear magnetic moment may be significant. In general, opposing spins (proton +1/2 and neutron −1/2) of the proton and the neutron cancel the magnetic field. (i) electron pairs with opposite spins cancel out magnetic moments, (ii) electron pair in an orbital cannot have parallel spins (Pauli's exclusion principle), (iii, iv and v): randomly oriented electrons do not result in net magnetism, (vii, viii and ix): electrons parallel to an applied field cause net magnetic moment.


 0 kommentar(er)
0 kommentar(er)
